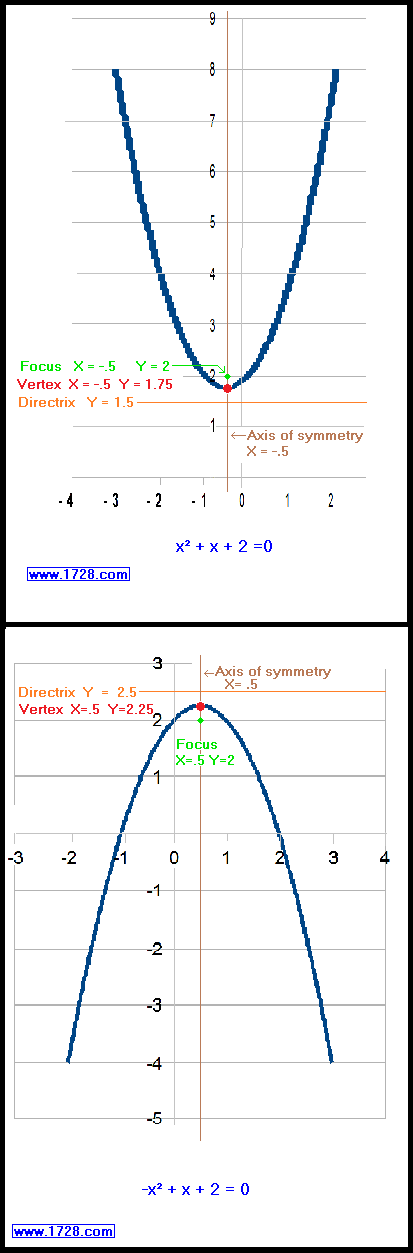
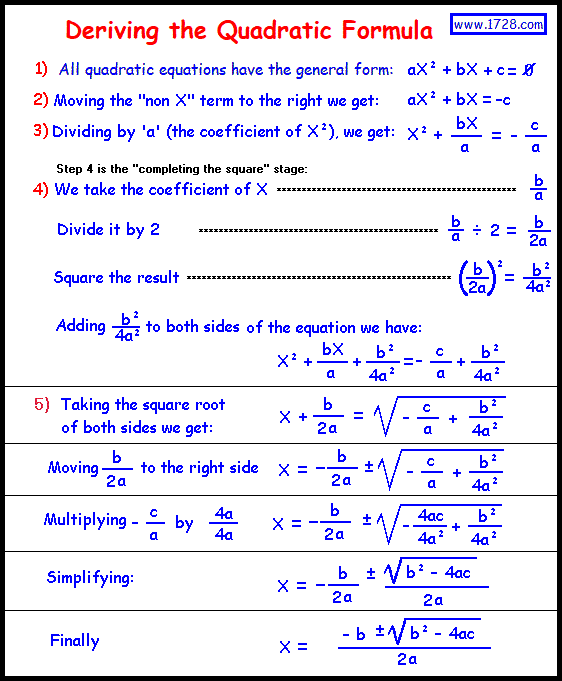
if the “a” coefficient is less than 0, the parabola is “∩-shaped”.When a quadratic equation is graphed, it forms a parabola.
A parabola is the locus of points equidistant from a
point called the focus and a line called the directrix.
Vertex
If “a” is greater than 0, the vertex of a parabola is the minimum point.
If “a” is less than 0, the vertex of a parabola is the maximum point.
The (x,y) point of the vertex is usually labelled as:
Vertex = (h, k)The “x” position (or “h”) is calculated with this formula:
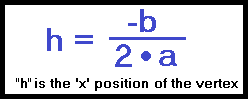
To get k, input the h value into this equation:
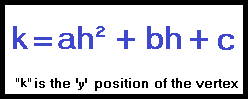
EXAMPLE
What is the vertex of the equation 2x2 -3x -4 =0?
h = -b ÷ (2•a)
h = –3 ÷ (2•2)
h = 3 ÷ 4
h = .75
k= 2•(h)2 -3•h -4
k= 2•(.75)2 -3•.75 -4
k= 2•(.75)2 -3•.75 -4
k= 1.125 -2.25 -4
k= -5.125
Vertex = (.75, -5.125)
The vertex is always halfway between the directrix and the focus.
Focus
The “x” value of a quadratic equation’s focus is the same as the “x” value of the vertex.
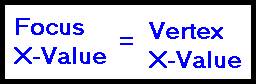
To find the “y” value of a quadratic equation’s focus we use the formula:
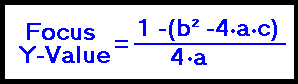
EXAMPLE
What is the focus of the equation 2x2 -3x -4 =0?
We already calculated the vertex “x” value as .75.
For the “y” value we calculate:
“y” value = (1 -(b2 -4 •a •c)) ÷ 4 •a
“y” value = (1 -(-32 -4 •2 •-4)) ÷ 4 •2
“y” value = (1 -(9 + 32)) ÷ 8
“y” value = 1 -41 ÷ 8
“y” value = -40 ÷ 8
“y” value of the focus = -5
Directrix
The equation for finding the y-value of the directrix is:
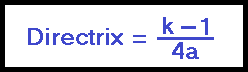
**************************************************************************
If you don’t want to use the formula, there is a simple way to find the “y” value of the directrix.
As stated before, the vertex is halfway between the directrix and the focus.
We’ve already calculated the “y” values of
the vertex (-5.125) and
the focus (-5)
The difference between these numbers is .125.
In the equation 2x2 -3x -4 =0, the “a” value is greater than zero so the directrix is below the vertex.
Therefore, the “y” value of the directrix is -5.125 minus .125 = -5.25.
(If this equation had a negative value of “a”, we would have added the .125 value because in that case, the directrix would be above the vertex).
As you probably have noticed, once you have calculated the focus or the vertex, just take the “x” value and you have the “x” value of the axis of symmetry.